Ans: C
Join $BC$ and add a point $D$ on $y$-axis such that $AB\perp CD$.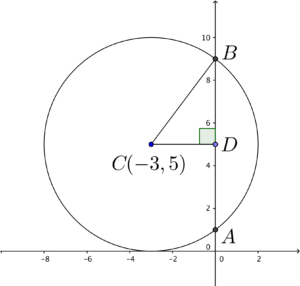
Join $BC$ and add a point $D$ on $y$-axis such that $AB\perp CD$.

Note that the distance between $CD$
$\begin{array}{cl}
= & 0 – (-3) \\
= & 3
\end{array}$
Since $CD \perp AB$, then $D$ is the mid-point of $AB$. i.e. $BD = 4$. By applying the Pythagoras Theorem to $\Delta BCD$, we have
$\begin{array}{rcl}
BC^2 & = & BD^2 + CD^2 \\
BC & = & \sqrt{4^2 + 3^2} \\
BC & = & 5
\end{array}$
Therefore, the equation of the circle $C$ is
$\begin{array}{rcl}
(x-(-3))^2 + (y-(5))^2 & = & (5)^2 \\
x^2 + 6x + 9 +y^2 -10y +25 & = & 25 \\
x^2 +y^2 +6x -10y +9 & = & 0
\end{array}$

