2014-I-17

$\begin{array}{rcl}
\dfrac{AB}{\sin \angle AVB} & = & \dfrac{VB}{\sin \angle VAB} \\
\dfrac{18}{\sin \angle AVB} & = & \dfrac{30}{\sin \angle 110^\circ} \\
\sin \angle AVB & = & \dfrac{18\sin 110^\circ}{30} \\
\angle AVB & = & 34.320\ 082\ 91^\circ
\end{array}$

所以，可得

$\begin{array}{rcl}
\angle VBA & = & 180^\circ – \angle AVB – \angle VAB \\
& = & 35.679\ 917\ 09^\circ \\
& \approx & 35.7^\circ
\end{array}$

$\begin{array}{rcl}
MP^2 & = & BP^2 + BM^2 -2(BP)(BM)\cos \angle VBA \\
MP^2 & = & 86.682\ 235\ 75 \\
MP & = & 9.310\ 329\ 519 \text{ cm}
\end{array}$

由於 $M$ 及 $N$ 分別為 $VB$ 及 $VC$ 的中點，在 $\Delta VBC$ 運用中點定理，可得

$\begin{array}{rcl}
MN & = & \dfrac{1}{2} \times BC \\
& = & 5 \text{ cm}
\end{array}$

由於 $P$ 及 $Q$ 分別為 $AB$ 及 $DC$ 的中點，則可得

$\begin{array}{rcl}
PQ & = & BC \\
& = & 10 \text{ cm}
\end{array}$

考慮梯形 $PQNM$。設 $h \text{ cm}$ 為梯形 $PQNM$ 的高。

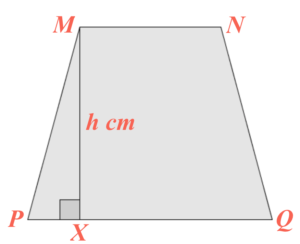
留意 $MP=NQ$，可得

$\begin{array}{rcl}
PX & = & \dfrac{PQ-MN}{2} \\
& = & 2.5\text{ cm}
\end{array}$

所以，可得

$\begin{array}{rcl}
h & = & \sqrt{MP^2 – PX^2} \\
& = & 8.968\ 402\ 073 \text{ cm}
\end{array}$

由此，梯形 $PQNM$ 的面積

$\begin{array}{cl}
= & \dfrac{1}{2} (MN+PQ)\times h \\
= & \dfrac{1}{2} ( 10+ 5 ) \times 8.968\ 402\ 073\\
= & 67.263\ 015\ 55 \text{ cm}^2 \\
< & 70 \text{ cm}^2 \end{array}$

所以，我同意該工匠。